LOG#050. Why riemannium?

Posted: 2012/11/07 | Author: amarashiki | Filed under: Physmatics, Zeta Zoology and polystuff | Tags: adelic identity, adelic ring, Astrophysics, atom, atomic physics, BE statistics, Berry-Keating conjecture, casimir effect, confinement, cosmological constant, Cosmology, Dirichlet eta function, FD statistics, fractals, group entropy, harmonic oscillator, hawking effect, Hilbert-Polya conjecture, logarithmic potentital, MB statistics, music, non-extensive entropy, Non-trivial Riemann zeroes, p-adic numbers, Paraboson, parafermion, Physmatics, prime numbers, QFT, Quantum chaos, quantum field theory, Quantum Mechanics, Quantum Statistics, random matrix theory, Riemann hypothesis, Riemann zeroes, Riemann zeta function, riemannium, schwinger effect, spectrum of riemannium, trivial Riemann zeroes, Tsallis statistics, Tsallisium, Veneziano amplitude, zeta values |7 Comments

DEDICATORY

1. THE RIEMANN ZETA FUNCTION ζ(s)

2. THE RIEMANN HYPOTHESIS

3. THE HILBERT-POLYA CONJECTURE

4. RANDOM MATRIX THEORY

5. QUANTUM CHAOS AND RIEMANN DYNAMICS

6. THE SPECTRUM OF RIEMANNIUM

7. ζ(s) AND RENORMALIZATION

8. ζ(s) AND QUANTUM STATISTICS

9. ζ(s) AND GROUP ENTROPIES

10. ζ(s) AND THE PRIMON GAS

11. LOG-OSCILLATORS

12. LOG-POTENTIAL AND CONFINEMENT

13. HARMONIC OSCILLATOR AND TSALLIS GAS

14. TSALLIS ENTROPIES IN A NUTSHELL

15. BEYOND QM/QFT: ADELIC WORLDS

16. STRINGS, FIELDS AND VACUUM

17. SUMMARY AND OUTLOOK

DEDICATORY

This special 50th log-entry is dedicated to 2 special people and scientists who inspired (and guided) me in the hard task of starting and writing this blog.

These two people are

1st. John C. Baez, a mathematical physicist. Author of the old but always fresh/brand new This Week Finds in Mathematical Physics, and now involved in the Azimuth blog. You can visit him here

http://johncarlosbaez.wordpress.com/

and here

http://math.ucr.edu/home/baez/

I was a mere undergraduate in the early years of the internet in my country when I began to read his TWF. If you have never done it, I urge to do it. Read him. He is a wonderful teacher and an excellent lecturer. John is now worried about global warming and related stuff, but he keeps his mathematical interests and pedagogical gifts untouched. I miss some topics about he used to discuss often before in his hew blog, but his insights about virtually everything he is involved into are really impressive. He also manages to share his entusiastic vision of Mathematics and Science. From pure mathematics to physics. He is a great blogger and scientist!

2nd. The professor Francis Villatoro. I am really grateful to him. He tries to divulge Science in Spain with his excellent blog ( written in Spanish language)

http://francisthemulenews.wordpress.com/

He is a very active person in the world of Spanish Science (and its divulgation). In his blog, he also tries to explain to the general public the latest news on HEP and other topics related with other branches of Physics, Mathematics or general Science. It is not an easy task! Some months ago, after some time reading and following his blog (as I do now yet, like with Baez’s stuff), I realized that I could not remain as a passive and simple reader or spectator in the web, so I wrote him and I asked him some questions about his experience with blogging and for advice. His comments and remarks were incredibly useful for me, specially during my first logs. I have followed several blogs the last years (like those by Baez or Villatoro), and I had no idea about what kind of style/scheme I should addopt here. I had only some fuzzy ideas about what to do, what to write and, of course, I had no idea if I could explain stuff in a simple way while keeping the physical intuition and the mathematical background I wanted to include. His early criticism was very helpful, so this post is a tribute for him as well. After all, he suggested me the topic of this post! I encourage you to read him and his blog (as long as you know Spanish or you can use a good translator).

Finally, let me express and show my deepest gratitude to John and Francis. Two great and extraordinary people and professionals in their respective fields who inspired (and yet they do) me in spirit and insight in my early and difficult steps of writing this blog. I am just convinced that Science is made of little, ordinary and small contributions like mine, and not only the greatest contributions like those making John and Francis to the whole world. I wish they continue making their contributions in the future for many, many years yet to come.

Now, let me answer the question Francis asked me to explain here with further details. My special post/log-entry number 50…It will be devoted to tell you why this blog is called The Spectrum of Riemannium, and what is behind the greatest unsolved problem in Number Theory, Mathematics and likely Physics/Physmatics as well…Enjoy it!

1. THE RIEMANN ZETA FUNCTION ζ(s)

The Riemann zeta function is a device/object/function related to prime numbers.

In general, it is a function of complex variable $s=\sigma+i\tau$ defined by the next equation:

$\boxed{\displaystyle{\zeta (s)=\sum_{n=1}^{\infty}n^{-s}=\sum_{n=1}^{\infty}\dfrac{1}{n^s}=\prod_{p=2}^{\infty}\dfrac{1}{1-p^{-s}}=\prod_{p,\; prime}\dfrac{1}{1-p^{-s}}}}$

$\boxed{\displaystyle{\zeta (s)=\dfrac{1}{1-2^{-s}}\dfrac{1}{1-3^{-s}}\ldots\dfrac{1}{1-137^{-s}}\ldots}}$

Generally speaking, the Riemann zeta function extended by analytical continuation to the whole complex plane is “more” than the classical Riemann zeta function that Euler found much before the work of Riemann in the XIX century. The Riemann zeta function for real and entire positive values is a very well known (and admired) series by the mathematicians. $\zeta (1)=\infty$ due to the divergence of the harmonic series. Zeta values at even positive numbers are related to the Bernoulli numbers, and it is still lacking an analytic expression for the zeta values at odd positive numbers.

The Riemann zeta function over the whole complex plane satisfy the following functional equation:

$\boxed{\pi^{-\frac{s}{2}}\Gamma \left(\dfrac{s}{2}\right)\zeta (s)=\pi^{-\frac{(1-s)}{2}}\Gamma \left(\dfrac{1-s}{2}\right)\zeta (1-s)}$

Equivalently, it can be also written in a very simple way:

$\boxed{\xi (s)=\xi (1-s)}$

where we have defined

$\xi (s)=\pi^{-\frac{s}{2}}\Gamma \left(\dfrac{s}{2}\right)\zeta (s)$

Riemann zeta values are an example of beautiful Mathematics. From $\displaystyle{\zeta (s)=\sum_{n=1}^{\infty}n^{-s}}$ , then we have:

1) $\zeta (0)=1+1+\ldots=-\dfrac{1}{2}$ .

2) $\zeta (1)=1+\dfrac{1}{2}+\dfrac{1}{3}+\ldots =\infty$ . The harmonic series is divergent.

3) $\zeta (2)=1+\dfrac{1}{2^2}+\dfrac{1}{3^2}+\ldots =\dfrac{\pi^2}{6}\approx 1.645$ . The famous Euler result.

4) $\zeta (3)=1+\dfrac{1}{2^3}+\dfrac{1}{3^3}+\ldots \approx 1.202$ . And odd zeta value called Apery’s constant that we do not know yet how to express in terms of irrational numbers.

5) $\zeta (4)=\dfrac{\pi^4}{90}\approx 1.0823$ .

6) $\zeta (-2n)=-\dfrac{\pi^{-n}}{2\Gamma (-n+1)}=0,\;\;\forall n=1,2,\ldots ,\infty$ . Trivial zeroes of zeta.

7) $\zeta (2n)=\dfrac{(-1)^{n+1}(2\pi)^{2n}B_{2n}}{2(2n)!}\;\;\forall n=1,2,\ldots ,\infty$ , where $B_{2n}$ are the Bernoulli numbers. The first 13 Bernoulli numbers are:

$B_0=1, B_1=-\dfrac{1}{2}, B_2=\dfrac{1}{6}, B_3=0, B_4=-\dfrac{1}{30}, B_5=0, B_6=\dfrac{1}{42}$

$B_7=0, B_8=-\dfrac{1}{30}, B_9=0, B_{10}=\dfrac{5}{66}, B_{11}=0, B_{12}=-\dfrac{691}{2730}, B_{13}=0$

8) We note that $B_{2n+1}=0,\;\; \forall n\geq 1$ .

9) $\zeta (-2n+1)=-\dfrac{B_{2n}}{2n}, \;\; \forall n=1,2,\ldots ,\infty$ .

For instance, $\zeta (-1)=-\dfrac{1}{12}=1+2+3+\ldots$ , $\zeta (-3)=\dfrac{1}{120}$ , and $\zeta (-5)=-\dfrac{1}{252}$ . Indeed, $\zeta (-1)$ arises in string theory trying to renormalize the vacuum energy of an infinite number of harmonic oscillators. The result in the bosonic string is $\dfrac{2}{2-D}$ . In order to match with Riemann zeta function regularization of the above series, the bosonic string is asked to live in an ambient spacetime of D=26 dimensions. We also have that

$\sum \vert n\vert^3=-\dfrac{1}{60}$

10) $\zeta (\infty)=1$ . The Riemann zeta value at the infinity is equal to the unit.

11) The derivative of the zeta function is $\displaystyle{\zeta '(s)=-\sum_{n=1}^{\infty}\dfrac{\log n}{n^s}}$ . Particularly important of this derivative are:

$\displaystyle{\zeta '(0)=-\sum_{n=1}^\infty \log n=-\log \prod_{n=1}^\infty n=\zeta (0)\log (2\pi)=-\dfrac{1}{2}\log (2\pi)=-\log \sqrt{2\pi}=\log \dfrac{1}{\sqrt{2\pi}}}$

or $\zeta '(0)=\log \sqrt{\dfrac{1}{2\pi}}$

This allow us to define the factorial of the infinity as

$\displaystyle{\infty !=\prod_{n=1}^{\infty}n=1\cdot 2\cdots \infty=e^{-\zeta '(0)}=\sqrt{2\pi}}$

and the renormalized infinite dimensional determinant of certain operator A as:

$\det _\zeta (A)=a_1\cdot a_2\cdots=\exp \left(-\zeta_A '(0)\right)$ , with $\displaystyle{\zeta _A (s)=\sum_{n=1}^\infty \dfrac{1}{a_n^s}}$

12) $\zeta (1+\varepsilon )=\dfrac{1}{\varepsilon}+\gamma_E +\mathcal{O} (\varepsilon )$ . This is a result used by theoretical physicists in dimensional renormalization/regularization. $\gamma_E\approx 0.577$ is the so-called Euler-Mascheroni constant.

The alternating zeta function, called Dirichlet eta function, provides interesting values as well. Dirichlet eta function is defined and related to the Riemann zeta fucntion as follows:

$\boxed{\displaystyle{\eta (s)=\sum_{n=1}^\infty \dfrac{(-1)^{n+1}}{n^s}=\left(1-2^{1-s}\right)\zeta (s)}}$

This can be thought as “bosons made of fermions” or “fermions made of bosons” somehow. Special values of Dirichlet eta function are given by:

$\eta (0)=-\zeta (0)=\dfrac{1}{2}$ $\eta (1)=\log 2$ $\eta (2)=\dfrac{1}{2}\zeta (2)=\dfrac{\pi^2}{12}$

$\eta (3)=\dfrac{3}{4}\zeta (3)\approx \dfrac{3}{4}(1.202)$ $\eta (4)=\dfrac{7}{8}\zeta (4)=\dfrac{7}{8}\left(\dfrac{\pi^4}{90}\right)$

Remark(I): $\zeta(2)$ is important in the physics realm, since the spectrum of the hydrogen atom has the following aspect

$E(n)=-\dfrac{K}{n^2}$

and the Balmer formula is, as every physicist knows

$\Delta E(n,m)=K\left(\dfrac{1}{n^2}-\dfrac{1}{m^2}\right)$

Remark (II): The fact that $\zeta (2)$ is finite implies that the energy level separation of the hydrogen atom in the Böhr level tends to zero AND that the sum of ALL the possible energy levels in the hydrogen atom is finite since $\zeta (2)$ is finite.

Remark(III): What about an “atom”/system with spectrum $E(n)=\kappa n^{-s}$ ? If $s=2$ , we do know that is the case of the Kepler problem. Moreover, it is easy to observe that $s=-1$ corresponds to tha harmonic oscillator, i.e., $E(n)=\hbar \omega n$ . We also know that $s=-2$ is the infinite potential well. So the question is, what about a $n^{-3}$ spectrum and so on?

In summary, does the following spectrum

$\boxed{E=\mathbb{K}\dfrac{1}{n^{s}}}$

with energy separation/splitting

$\boxed{\Delta E(n,m;s)=\mathbb{K}\left(\dfrac{1}{n^{s}}-\dfrac{1}{m^{s}}\right)}$

exist in Nature for some physical system beyond the infinite potential well, the harmonic oscillator or the hydrogen atom, where $s=-2$ , $s=-1$ and $s=2$ respectively?

It is amazing how Riemann zeta function gets involved with a common origin of such a different systems and spectra like the Kepler problem, the harmonic oscillator and the infinite potential well!

2. THE RIEMANN HYPOTHESIS

The Riemann Hypothesis (RH) is the greatest unsolved problem in pure Mathematics, and likely, in Physics too. It is the statement that the only non-trivial zeroes of the Riemann zeta function, beyond the trivial zeroes at $s=-2n,\;\forall n=1,2,\ldots,\infty$ have real part equal to 1/2. In other words, the equation or feynmanity has only the next solutions:

$\boxed{\mbox{RH:}\;\;\zeta (s)=0\leftrightarrow \begin{cases} s_n=-2n,\;\forall n=1,\ldots,\infty\;\;\mbox{Trivial zeroes}\\ s_n=\dfrac{1}{2}\pm i\lambda_n, \;\;\forall n=1,\ldots,\infty \;\;\mbox{Non-trivial zeroes}\end{cases}}$

I generally prefer the following projective-like version of the RH (PRH):

$\boxed{\mbox{PRH:}\;\;\zeta (s)=0\leftrightarrow \begin{cases} s_n=-2n,\;\forall n=1,\ldots,\infty\;\;\mbox{Trivial zeroes}\\ s_n=\dfrac{1\pm i\overline{\lambda}_n}{2}, \;\;\forall n=1,\ldots,\infty \;\;\mbox{Non-trivial zeroes}\end{cases}}$

The Riemann zeta function can be sketched on the whole complex plane, in order to obtain a radiography about the RH and what it means. The mathematicians have studied the critical strip with ingenious tools an frameworks. The now terminated ZetaGrid project proved that there are billions of zeroes IN the critical line. No counterexample has been found of a non-trivial zeta zero outside the critical line (and there are some arguments that make it very unlikely). The RH says that primes “have music/order/pattern” in their interior, but nobody has managed to prove the RH. The next picture shows you what the RH “say” graphically:

If you want to know how the Riemann zeroes sound, M. Watkins has done a nice audio file to see their music.

You can learn how to make “music” from Riemann zeroes here http://empslocal.ex.ac.uk/people/staff/mrwatkin/zeta/munafo-zetasound.htm

And you can listen their sound here

http://empslocal.ex.ac.uk/people/staff/mrwatkin/zeta/zeta.mp3

Riemann zeroes are connected with prime numbers through a complicated formula called “the explicit formula”. The next equation holds $\forall x\geq 2$ integer numbers, and non-trivial Riemann zeroes in the complex (upper) half-plane with $\tau>0$ :

$\boxed{\displaystyle{\pi (x)+\sum_{n=2}^\infty \dfrac{\pi \left( x^{1/n}\right)}{n}=\text{Li} (x)-\sum_{\lambda =\sigma+i\tau }\left(\text{Li}(x^\lambda)+\text{Li}\left( x^{1-\lambda}\right)\right)+\int_x^\infty\dfrac{du}{u(u^2-1)\ln u}-\ln 2}}$

and where $\pi (x)$ is the celebrated Gauss prime number counting function, i.e., $\pi (x)$ represents the prime numbers that are equal than x or below. This explicit formula was proved by Hadamard. The explicit formula follows from both product representations of $\zeta (s)$ , the Euler product on one side and the Hadamard product on the other side.

The function $\text{Li} (x)$ , sometimes written as $\text{li} (x)$ , is the logarithmic integral

$\displaystyle{\text{Li} (x) =\text{li} (x)= \int_2^x\dfrac{du}{\ln x}}$

The explicit formula comes in some cool variants too. For instance, we can write

$\pi (x)=\pi_0 (x)+\pi_1 (x)=\pi_{\mbox{smooth}}+\pi_{\mbox{osc-chaotic}}$

where

$\displaystyle{\pi_0 (x)=\sum_{n=1}^\infty\dfrac{\mu (n)}{n}\left[\mbox{Li}(x^{1/n})-\sum_{k=1}^\infty\mbox{Li}(x^{-2k/n})\right]}$

and

$\displaystyle{\pi_1 (x)=-2\mbox{Re}\sum_{n=1}^\infty\dfrac{\mu (n)}{n}\sum_{\alpha=1}^\infty\mbox{Li}(x^{(\sigma_\alpha+i\tau_\alpha)/n})}$

For large values of x, we have the asymptotics

$\pi_0 (x)\approx \mbox{Li} (x)$

and

$\displaystyle{\pi_1 (x)\approx -\dfrac{2}{\ln x}\sum_{\alpha=1}^\infty\dfrac{x^{\sigma_\alpha}}{\sigma_\alpha^2+\tau_\alpha^2}\left(\sigma_\alpha\cos (\tau_\alpha \ln x)+\tau_\alpha \sin (\tau_\alpha \ln x)\right)}$

Remark: Please, don’t confuse the logarithmic integral with the polylogarithm function $\text{Li}_x (s)$ .

Gauss also conjectured that

$\pi (x)\sim \text{Li} (x)$

3. THE HILBERT-POLYA CONJECTURE

Date: January 3, 1982. Andrew Odlyzko wrote a letter to George Pólya about the physical ground/basis of the Riemann Hypothesis and the conjecture associated to Polya himself and David Hilbert. Polya answered and told Odlyzko that while he was in Göttingen around 1912 to 1914 he was asked by Edmund Landau for a physical reason that the Riemann Hypothesis should be true, and suggested that this would be the case if the imaginary parts, say $T$ of the non-trivial zeros

$\dfrac{1}{2}+iT$

of the Riemann zeta function corresponded to eigenvalues of an unbounded and unknown self adjoint operator $\hat{T}$ . That statement was never published formally, but it was remembered after all, and it was transmitted from one generation to another. At the time of Pólya’s conversation with Landau, there was little basis for such speculation. However, Selberg, in the early 1950s, proved a duality between the length spectrum of a Riemann surface and the eigenvalues of its Laplacian. This so-called Selberg trace formula shared a striking resemblance to the explicit formula of certain L-function, which gave credibility to the speculation of Hilbert and Pólya.

4. RANDOM MATRIX THEORY

Dialogue(circa 1970). “(…)Dyson: So tell me, Montgomery, what have you been up to? Montgomery: Well, lately I’ve been looking into the distribution of the zeros of the Riemann zeta function. Dyson: Yes? And? Montgomery: It seems the two-point correlations go as….(…) Dyson: Extraordinary! Do you realize that’s the pair-correlation function for the eigenvalues of a random Hermitian matrix? It’s also a model of the energy levels in a heavy nucleus, say U-238.(…)”

A step further was given in the 1970s, by the mathematician Hugh Montgomery. He investigated and found that the statistical distribution of the zeros on the critical line has a certain property, now called Montgomery’s pair correlation conjecture. The Riemann zeros tend not to cluster too closely together, but to repel. During a visit to the Institute for Advanced Study (IAS) in 1972, he showed this result to Freeman Dyson, one of the founders of the theory of random matrices. Dyson realized that the statistical distribution found by Montgomery appeared to be the same as the pair correlation distribution for the eigenvalues of a random and “very big/large” Hermitian matrix with size NxN. These distributions are of importance in physics and mathematics. Why? It is simple. The eigenstates of a Hamiltonian, for example the energy levels of an atomic nucleus, satisfy such statistics. Subsequent work has strongly borne out the connection between the distribution of the zeros of the Riemann zeta function and the eigenvalues of a random Hermitian matrix drawn from the theoyr of the so-calle Gaussian unitary ensemble, and both are now believed to obey the same statistics. Thus the conjecture of Pólya and Hilbert now has a more solid fundamental link to QM, though it has not yet led to a proof of the Riemann hypothesis. The pair-correlation function of the zeros is given by the function:

$R_2(x)=1-\left(\dfrac{\sin \pi x}{\pi x}\right)^2$

In a posterior development that has given substantive force to this approach to the Riemann hypothesis through functional analysis and operator theory, the mathematician Alain Connes has formulated a “trace formula” using his non-commutative geometry framework that is actually equivalent to certain generalized Riemann hypothesis. This fact has therefore strengthened the analogy with the Selberg trace formula to the point where it gives precise statements. However, the mysterious operator believed to provide the Riemann zeta zeroes remain hidden yet. Even worst, we don’t even know on which space the Riemann operator is acting on.

However, some trials to guess the Riemann operator has been given from a semiclassical physical environtment as well. Michael Berry and Jon Keating have speculated that the Hamiltonian/Riemann operator $H$ is actually some kind of quantization of the classical Hamiltonian $XP$ where $P$ is the canonical momentum associated with the position operator $X$ . If that Berry-Keating conjecture is true. The simplest Hermitian operator corresponding to $XP$ is

$H = \dfrac1{2} (xp+px) = - i \left( x \dfrac{\mathrm{d}}{\mathrm{d} x} + \dfrac{1}{2} \right)$

At current time, it is still quite inconcrete, as it is not clear on which space this operator should act in order to get the correct dynamics, nor how to regularize it in order to get the expected logarithmic corrections. Berry and Germán Sierra, the latter in collaboration with P.K.Townsed, have conjectured that since this operator is invariant under dilatations perhaps the boundary condition $f(nx)=f(x)$ for integer $n$ may help to get the correct asymptotic results valid for big $n$ . That it, in the large $n$ we should obtain

$s_n=\dfrac{1}{2} + i \dfrac{ 2\pi n}{\log n}$

5. QUANTUM CHAOS AND RIEMANN DYNAMICS

Indeed, the Berry-Keating conjecture opened another striking attack to prove the RH. A topic that was popular in the 80’s and 90’s in the 20th century. The weird subject of “quantum chaos”. Quantum chaos is the subject devoted to the study of quantum systems corresponding to classically chaotic systems. The Berry-Keating conjecture shed light further into the Riemann dynamics, sketching some of the properties of the dynamical system behind the Riemann Hypothesis.

In summary, the dynamics of the Riemann operator should provide:

1st. The quantum hamiltonian operator behind the Riemann zeroes, in addition to the classical counterpart, the classical hamiltonian $H$ , has a dynamics containing the scaling symmetry. As a consequence, the trajectories are the same at all energy scale.
2nd. The classical system corresponding to the Riemann dynamics is chaotic and unstable.
3rd. The dynamics lacks time-reversal symmetry.
4th. The dynamics is quasi one-dimensional.

A full dictionary translating the whole correspondence between the chaotic system corresponding to the Riemann zeta function and its main features is presented in the next table:

6. THE SPECTRUM OF RIEMANNIUM

In 2001, the following paper emerged, http://arxiv.org/abs/nlin/0101014. The Riemannium arxiv paper was published later (here: Reg. Chaot. Dyn. 6 (2001) 205-210). After that, Brian Hayes wrote a really beautiful, wonderful and short paper titled The Spectrum of Riemannium in 2003 (American Scientist, Volume 91, Number 4 July–August, 2003,pages 296–300). I remember myself reading the manuscript and being totally surprised. I was shocked during several weeks. I decided that I would try to understand that stuff better and better, and, maybe, make some contribution to it. The Spectrum of Riemannium was an amazing name, an incredible concept. So, I have been studying related stuff during all these years. And I have my own suspitions about what the riemannium and the zeta function are, but this is not a good place to explain all of them!

The riemannium is the mysterious physical system behind the RH. Its spectrum, the spectrum of riemannium, are given by the RH and its generalizations.

Moreover, the following sketch from Hayes’ paper is also very illustrative:

What do you think? Isn’t it suggestive? Is it amazing?

7. ζ(s) AND RENORMALIZATION

Riemann zeta function also arises in the renormalization of the Standard Model and the regularization of determinants with “infinite size” (i.e., determinants of differential operators and/or pseudodifferential operators). For instance, the $\infty$ -dimensional regularized determinant is defined through the Riemann zeta function as follows:

$\displaystyle{\det _\zeta \mathcal{P}=e^{-\zeta_{\mathcal{P}}^{'}(0)}}$

The dimensional renormalization/regularization of the SM makes use of the Riemann zeta function as well. It is ubiquitous in that approach, but, as far as I know, nobody has asked why is that issue important, as I have suspected from long time ago.

8. ζ(s) AND QUANTUM STATISTICS

Riemann zeta function is also used in the theory of Quantum Statistics. Quantum Statistics are important in Cosmology and Condensed Matter, so it is really striking that Riemann zeta values are related to phenomena like Bose-Einstein condensation or the Cosmic Microwave Background and also the yet to be found Cosmic Neutrino Background!

Let me begin with the easiest quantum (indeed classical) statistics, the Maxwell-Boltzmann (MB) statistics. In 3 spatial dimensions (3d) the MB distribution arises ( we will work with units in which $\hbar =1$ ):

$f(p)_{MB}=\dfrac{1}{(2\pi)^3}e^{\frac{\mu -E}{k_BT}}$

Usually, there are 3 thermodynamical quantities that physicists wish to compute with statistical distributions: 1) the number density of particles $n=N/V$ , 2) the energy density $\varepsilon=U/V$ and 3) the pressure $P$ . In the case of a MB distribution, we have the following definitions:

$\displaystyle{n=\dfrac{1}{(2\pi)^3}\int d^3p e^{\frac{\mu -E}{k_BT}}}$

$\displaystyle{\varepsilon =\dfrac{1}{(2\pi)^3}\int d^3p Ee^{\frac{\mu -E}{k_BT}}}$

$\displaystyle{\varepsilon =\dfrac{1}{(2\pi)^3}\int d^3p \dfrac{1}{3}\dfrac{\vert\mathbf{p}\vert^2}{E}e^{\frac{\mu -E}{k_BT}}}$

We can introduce the dimensionless variables $late z=\dfrac{mc^2}{k_BT}$, $\tau =\dfrac{E}{k_BT}=\dfrac{\sqrt{p^2+m^2c^4}}{k_BT}$ . In this way,

$\vert p\vert=\dfrac{k_BT}{c}\sqrt{\tau^2-z^2}$

$c^2\vert\mathbf{p}\vert d\vert \mathbf{p}\vert=k_B^2T^2\tau d\tau$

$c^3\vert\mathbf{p}\vert^2d\vert\mathbf{p}\vert=k_B^3T^3\tau\sqrt{\tau^2-z^2}d\tau$

With these definitions, the particle density becomes

$\displaystyle{n=\dfrac{4\pi k_B^3T^3}{(2\pi)^3}e^{\frac{\mu}{k_BT}}\int_z^\infty d\tau (\tau^2-z^2)^{1/2}\tau e^{-\tau}}$

This integral can be calculated in closed form with the aid of modified Bessel functions of the 2th kind:

$K_n (z)=\dfrac{2^nn!}{(2n)!z^n}\int_z^\infty d\tau (\tau^2-z^2)^{n-1/2}e^{-\tau}$ or equivalently

$K_n (z)=\dfrac{2^{n-1}(n-1)!}{(2n-2)!z^n}\int_z^\infty d\tau (\tau^2-z^2)^{n-3/2}\tau e^{-\tau}$

$K_{n+1} (z)=\dfrac{2nK_n (z)}{z}+K_{n-1} (z)$

$\displaystyle{K_2 (x)=\dfrac{1}{z^2}\int_z^\infty (\tau^2-z^2)^{1/2}\tau e^{-\tau}d\tau}$

And thus, we have the next results (setting $c=1$ for simplicity):

$\mbox{Particle number density}\equiv n=\dfrac{N}{V}=\dfrac{k_B^3T^3}{2\pi^2}z^2K_2 (z)=\dfrac{k_B^3T^3}{2\pi^2}\left(\dfrac{m}{k_BT}\right)^2K_2\left(\dfrac{m}{k_BT}\right)e^{\frac{\mu}{k_BT}}$

$\mbox{Energy density}\equiv\varepsilon=\dfrac{k_B^4T^4}{2\pi^2}\left[ 3\left(\dfrac{m}{k_BT}\right)^2K_2\left(\dfrac{m}{k_BT}\right)+\left(\dfrac{m}{k_BT}\right)^3K_1\left(\dfrac{m}{k_BT}\right)\right]e^{\frac{\mu}{k_BT}}$

$\mbox{Pressure}\equiv P=\dfrac{k_B^4T^4}{2\pi^2}\left(\dfrac{m}{k_BT}\right)^2K_2\left(\dfrac{m}{k_BT}\right)e^{\frac{\mu}{k_BT}}$

Even entropy density is easiy to compute:

$\mbox{Entropy density}\equiv s=\dfrac{m^3}{2\pi^2}e^{\frac{\mu}{k_BT}}\left[ K_1\left(\dfrac{m}{k_BT}\right)+\dfrac{4k_BT-\mu}{m}K_2\left(\dfrac{m}{k_BT}\right)\right]$

These results can be simplified in some limit cases. For instance, in the massless limit $z=m/k_BT\rightarrow 0$ . Moreover, we also know that $\displaystyle{\lim_{z\rightarrow 0}z^nK_n (z)=2^{n-1}(n-1)!}$ . In such a case, we obtain:

$n\approx \dfrac{k_B^3T^3}{\pi^2}e^{\frac{\mu}{k_BT}}$

$\varepsilon \approx \dfrac{3k_B^4T^4}{\pi^2}e^{\frac{\mu}{k_BT}}$

$P\approx \dfrac{k_B^4T^4}{\pi^2}e^{\frac{\mu}{k_BT}}$

We note that $\varepsilon=3P$ in this massless limit.

Remark (I): In the massless limit, and whenever there is no degeneracy, $\varepsilon =3P$ holds.

Remark (II): If there is a quantum degeneracy in the energy levels, i.e., if $g\neq 1$ , we must include an extra factor of $g_j=2j+1$ for massive particles of spin j. For massless photons with helicity, there is a $g=2$ degeneracy.

Remark (III): In the D-dimensional (D=d+1) Bose gas with dispersion relationship $\varepsilon_p=cp^{s}$ , it can be shown that the pressure is related with the energy density in the following way

$\mbox{Pressure}\equiv P=\dfrac{s}{d}\dfrac{U}{V}=\dfrac{s}{d}\varepsilon$

Remark (IV): Let us define $p^s (n)$ as the number of ways an integer number can be expressed as a sum of the sth powers of integers. For instance,

$p^1 (5)=7$ because $5=4+1=3+2=3+1+1=2+2+1=2+1+1+1=1+1+1+1+1$

$p^2 (5)=2$ because $5=2^2+1^2=1^2+1^2+1^2+1^2+1^2$

If $E_n=n^s$ with $n\geq 1$ and $s>0$ , then $x=e^{-\beta}$ and the partition function is

$\displaystyle{Z=\prod_{k}\left( 1+e^{\frac{\mu-E}{k_BT}}\right)}$

We will see later that $\displaystyle{\sum_{N=0}^\infty x^N=\begin{cases}1+x, FD \\ \dfrac{1}{1-x}, BE\end{cases}}$

with $\mu =0$ is nothing but the generatin function of the partitions $p^s (n)$

$\displaystyle{Z(x=e^{-\beta})=\prod_{n=1}^\infty \dfrac{1}{1-x^{n^s}}=\sum_{n=1}^\infty p^s (n) x^n\approx \int_1^\infty dn p^s (n) e^{-\beta n}}$

The Hardy-Ramanujan inversion formula reads (for the case s=1 only):

$p(n) \approx \dfrac{1}{4\sqrt{3}N}e^{\pi\sqrt{2N/3}}$

Remark (V): There are some useful integrals in quantum statistics. They are the so-called Bose-Einstein/Fermi-Dirac integrals

$\displaystyle{\int_0^\infty dx \dfrac{x^{n-1}}{e^x\mp 1}=\begin{cases}\Gamma (n) \zeta (n), \;\; BE\\ \Gamma (n)\eta (n)=\Gamma (n) (1-2^{1-n})\zeta (n),\;\; FD\end{cases}}$

The BE-FD quantum distributions in 3d are defined as follows:

$\displaystyle{f(p)=\dfrac{1}{(2\pi)^3}\sum_{n=1}^{\infty}(\mp)^{n+1}e^{-n\frac{(E-\mu)}{k_BT}}}$

where the minus sign corresponds to FD and the plus sign to BE.

We will firstly study the BE distribution in 3d. We have:

$\displaystyle{n=\dfrac{1}{(2\pi)^3}\int d^3p \left(e^{\frac{\mu-E}{k_BT}}-1\right)^{-1}=\dfrac{1}{(2\pi)^3}\int d^3p \sum_{n=1}^{\infty}(+1)^{n+1}e^{\frac{n\mu-nE}{k_BT}}}$

Introducing a scaled temperature $T'=T/n$ , we get

$\displaystyle{n=\sum_{n=1}^{\infty}\left[\dfrac{1}{(2\pi)^3}\int d^3p e^{\frac{n\mu-nE}{k_BT'}}\right]=\sum_{n=1}^{\infty}\dfrac{k_B^3T^3}{2\pi^2}\dfrac{1}{n^3}\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)e^{\frac{n\mu}{k_BT}}}$

$\displaystyle{\varepsilon=\sum_{n=1}^{\infty}\dfrac{k_B^4T^4}{n^4(2\pi^2)}\left[3\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)+\left(\dfrac{nm}{k_BT}\right)^3K_1\left(\dfrac{nm}{k_BT}\right)\right]e^{\frac{n\mu}{k_BT}}}$

$\displaystyle{P=\sum_{n=1}^{\infty}\dfrac{k_B^4T^4}{n^4(2\pi^2)}\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)e^{\frac{n\mu}{k_BT}}}$

Again, we can study a particularly simple case: the massless limit $m\rightarrow 0$ with $\mu\rightarrow 0$ . In this case, we get:

$\displaystyle{n=\dfrac{k_B^3T^3}{\pi^2}\sum_{n=1}^\infty \dfrac{1}{n^3}=\dfrac{k_B^3T^3}{\pi^2}\zeta (3)\approx 1.202\dfrac{k_B^3T^3}{\pi^2}}$

$\displaystyle{\varepsilon=\sum_{n=1}^\infty\dfrac{3(k_BT)^4}{\pi^2}\dfrac{1}{n^4}=\dfrac{3(k_BT)^4\zeta (4)}{\pi^2}=\dfrac{\pi^2}{30}(k_BT)^4}$

$\displaystyle{P=\sum_{n=1}^\infty\dfrac{(k_BT)^4}{\pi^2}\dfrac{1}{n^4}=\dfrac{(k_BT)^4\zeta (4)}{\pi^2}=\dfrac{\pi^2(k_BT)^4}{90}}$

The FD distribution in 3d can be studied in a similar way. Following the same approach as the BE distribution, we deduce that:

$\displaystyle{n=\sum_{n=1}^\infty (-1)^{n+1}\dfrac{(k_BT)^3}{2\pi^2n^3}\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)e^{\frac{\mu n}{k_BT}}}$

$\displaystyle{\varepsilon= \sum_{n=1}^\infty (-1)^{n+1}\dfrac{(k_BT)^4}{2\pi^2}\left[3\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)+\left(\dfrac{nm}{k_BT}\right)^3K_1\left(\dfrac{nm}{k_BT}\right)\right]e^{\frac{\mu n}{k_BT}}}$

$\displaystyle{P=\sum_{n=1}^\infty (-1)^{n+1}\dfrac{(k_BT)^4}{2\pi^2}\dfrac{1}{n^4}\left(\dfrac{nm}{k_BT}\right)^2K_2\left(\dfrac{nm}{k_BT}\right)e^{\frac{n\mu}{k_BT}}}$

and again the massless limit $m=0$ and $\mu\rightarrow 0$ provide

$\displaystyle{n\approx \dfrac{(k_BT)^3}{\pi^2}\sum_{n=1}^\infty (-1)^{n+1}\dfrac{1}{n^3}=\dfrac{(k_BT)^3}{\pi^2}\eta (3)=\dfrac{(k_BT)^3}{\pi^2}\left(\dfrac{3}{4}\right)\zeta (3)}$

$\displaystyle{\varepsilon\approx \dfrac{3(k_BT)^4}{\pi^2}\sum_{n=1}^\infty (-1)^{n+1}\dfrac{1}{n^4}=3(k_BT)^4\eta (4)=3(k_BT)^4\dfrac{7}{8}\zeta (4)=\dfrac{\pi^2(k_BT)^4}{30}\left(\dfrac{7}{8}\right)}$

$\displaystyle{P\approx \dfrac{(k_BT)^4}{\pi^2}\sum_{n=1}^\infty (-1)^{n+1}\dfrac{1}{n^4}=\left(\dfrac{7}{8}\right)\dfrac{\pi^2(k_BT)^4}{90}}$

Remark (I): For photons $\gamma$ with degeneracy $g=2$ we obtain

$n_\gamma =\dfrac{2\zeta (3) (k_BT)^3}{\pi^2}$

$\varepsilon_\gamma= 3P_\gamma =\dfrac{\pi^2 (k_BT)^4}{15}$

$s_\gamma =P'(T)=\dfrac{4}{3}\left(\dfrac{\pi^2}{15}\right)(k_BT)^3=\dfrac{2\pi^4}{45\zeta (3)}n$

Remark (II): In Cosmology, Astrophysics and also in High Energy Physics, the following units are used

$1eV=1.602\cdot 10^{-19}J$

$\hbar=1=6.58\cdot 10^{-22}MeVs=7.64\cdot 10^{-12}Ks$

$\hbar c=1=0.19733GeV\cdot fm=0.2290 K\cdot cm$

$1 K=0.1532\cdot 10^{-36}g\cdot c^2$

The Cosmic Microwave Background is the relic photon radiation of the Big Bang, and thus it has a temperature due to photons in the microwave band of the electromagnetic spectrum. Its value is:

$T_\gamma \approx 2.725K$

Indeed, it also implies that the relic photon density is about $n_\gamma =410\dfrac{1}{cm^3}$

It is also speculated that there has to be a Cosmic Neutrino Background relic from the Big Bang. From theoretical Cosmology, it is related to the photon CMB temperature in the following way:

$T_\nu =\left(\dfrac{4}{11}\right)^{1/3}2.7K$ or equivalently

$T_\nu\approx 1.9K$

This temperature implies a relic neutrino density (per species, i.e., with $g_\nu=1$ ) about

$n_\nu=54\dfrac{1}{cm^3}$

The cosmological density entropy due to these particles is

$s_0=\dfrac{S_0}{V}=\dfrac{4\pi^2}{45}\left[1+\dfrac{2\cdot 3}{2}\left(\dfrac{7}{8}\right)\left(\dfrac{4}{11}\right)\right]T_{0\gamma}^3=2810\dfrac{1}{cm^3}\left( \dfrac{T_{0\gamma}}{2.7K}\right)^3$

and then we get

$s_0\approx 7.03n_{0\gamma}$

Remark (III): In Cosmology, for fermions in 3d ( note that BE implies $\varepsilon=3P$ , and that we must drop the factors $\left( 7/8\right), \left( 3/4\right), \left( 7/6\right)$ in the next numerical values) we can compute

$n=\begin{cases}\left(\dfrac{g}{2}\right)\left(\dfrac{3}{4}\right)\dfrac{2\zeta (3)}{\pi^2}(k_BT)^3\\ \left(\dfrac{g}{2}\right)\left(\dfrac{3}{4}\right)31.700\left(\dfrac{k_BT}{GeV}\right)^3\dfrac{1}{fm^3}\\ \left(\dfrac{g}{2}\right)\left(\dfrac{3}{4}\right)20.288\left(\dfrac{T}{K}\right)^3\dfrac{1}{cm^3}\end{cases}$

$\varepsilon=3P=\begin{cases}\left(\dfrac{g}{2}\right)\left(\dfrac{7}{8}\right)\left(\dfrac{\pi^2}{15}\right)(k_BT)^4\\ \left(\dfrac{g}{2}\right)\left(\dfrac{7}{8}\right)(85.633)\left(\dfrac{k_BT}{GeV}\right)\dfrac{GeV}{fm^3}\\ \left(\dfrac{g}{2}\right)\left(\dfrac{7}{8}\right)\left(0.841\cdot 10^{-36}\right)\left(\dfrac{T}{K}\right)^4\dfrac{g}{cm^3}\end{cases}$

$s=\dfrac{S}{V}=\left(\dfrac{g}{2}\right)\left(\dfrac{7}{8}\right)\left(\dfrac{4\pi^2}{45}\right)(k_BT)^3=\dfrac{7}{6}\left[\dfrac{2\pi^4}{45\zeta (3)}\right] n$

Remark (IV): An example of the computation of degeneracy factor is the quark-gluon plasma degeneracy $g_{QGP}$ . Firstly we compute the gluon and quark degeneracies

$g_g=(\mbox{color})(\mbox{spin})=2^3\cdot 2=8\cdot 2=16$

$g_q=(p\overline{p})(\mbox{spin})(\mbox{color})(\mbox{flavor})=2\cdot 2\cdot 3\cdot N_f=12N_f$

Then, the QG plasma degeneracy factor is

$g_{QGP}=g_g+\dfrac{7}{8}g_q=16+\dfrac{7}{8}12N_f=16+\dfrac{21}{2}N_f \leftrightarrow \boxed{g_{QGP}=16+\dfrac{21}{2}N_f}$

In general, for charged leptons and nucleons $g=2$ , $g=1$ for neutrinos (per species, of course), and $g=2$ for gluons and photons. Remember that massive particles with spin j will have $g_j=2j+1$ .

Remark (V): For the Planck distribution, we also get the known result for the thermal distribution of the blackbody radiation

$\displaystyle{I(T)=\int_0^\infty f(\nu ,T)d\nu=\dfrac{8\pi h}{c^3}\int_0^\infty \dfrac{\nu^3d\nu}{e^{\frac{h\nu}{k_BT}}-1}=\dfrac{8\pi^5k_B^4T^4}{15c^3h^3}}$

Remark (VI): Sometimes the following nomenclature is used

i) Extremely degenerated gas if $\mu>>k_BT$

ii) Non-degenerated gas if $\mu <<-k_BT$

iii) Extremely relativistic gas ( or ultra-relativistic gas) if $p>> mc$

iv) Non-relativistic gas if $p<<mc$

9. ζ(s) AND GROUP ENTROPIES

Let us define the following shift operator $\hat{T}$ :

$\hat{T}f(x)=f(x+\sigma)$

where $\sigma\in \mathbb{R}$ . Moreover, there is certain isomorphism between the shift operator space and the space of functions through the map $\hat{T}\leftrightarrow x^\sigma$ .

We define the generalized logarithm as the image under the previous map of $\hat{T}$ . That is:

$\displaystyle{\mbox{Log}_G(x)\equiv \dfrac{1}{\sigma}\sum_{n=l}^{m}k_n x^{\sigma n}}$

where $l,m\in \mathbb{Z}$ , with $l<m$ , $m-l=r$ and $x>0$ . Furthermore, the next contraints are also given for every generalized logarithm:

1st. $\displaystyle{\sum_{n=1}^m k_n=0}$ .

2nd. $\displaystyle{\sum_{n=l}^m nk_n=c}$ , $k_m\neq 0$ , and $k_l\neq 0$ .

3rd. $\displaystyle{\sum_{n=l}^m\vert n\vert^l k_n=K_l}$ , $\forall l=2,3,\ldots ,m-l$ and where $K_l \in \mathbb{R}$ .

With these definitions we also have that

A) $\mbox{Log}_G(x)=\ln (x)$

B) $\mbox{Log}_G(1)=0$

Examples of generalized logarithms are:

1) The Tsallis logarithm.

$\mbox{Log}_T(x)=\dfrac{x^{1-q}-1}{1-q}$

2) The Kaniadakis logarithm.

$\mbox{Log}_K(x)=\dfrac{x^\kappa-x^{-\kappa}}{2\kappa}$

3) The Abe logarithm.

$\mbox{Log}_A(x)=\dfrac{x^{\sigma -1}-x^{\sigma^{-1}-1}}{\sigma-\sigma^{-1}}$

4) The biparametric logarithm.

$\mbox{Log}_B(x)=\dfrac{x^a-x^b}{a-b}$

with $a=\sigma-1$ and $b=\sigma^{-1}-1$ in the case of the Abe logarithm.

Group entropies are defined through the use of generalized logarithms. Define some discrete probability distribution $\left[ p_i\right]_{i=1,\ldots,W}$ with normalization $\displaystyle{\sum_{i=1}^Wp_i=1}$ . Therefore, the group entropy is the following functional sum:

$\boxed{\displaystyle{S_G=-k_B\sum_{i=1}^{W}p_i \mbox{Log}_G \left(\dfrac{1}{p_i}\right)}}$

where we have used the previous definition of generalized logarithm and the Boltzmann’s constant $k_B$ is a real number. It is called group entropy due to the fact that $S_G$ is connected to some universal formal group. This formal group will determine some correlations for the class of physical systems under study and its invariant properties. In fact, the Tsallis logarithm itself is related to the Riemann zeta function through a beautiful equation! Under the Tsallis group exponential, the isomorphism $x\leftrightarrow e^t$ is defined to be $e_G^t=\dfrac{e^{(1-q)t}-1}{1-q}$ , and thus we easily get:

$\displaystyle{\dfrac{1}{\Gamma (s)}=\int_0^\infty\dfrac{1}{\dfrac{e^{(1-q)t}-1}{1-q}}t^{s-1}dt=\dfrac{\zeta (s)}{(1-q)^{s-1}}}$

$\forall s$ such as $Re (s)>1$ and $q<1$ .

10. ζ(s) AND THE PRIMON GAS

The primon gas/free Riemann gas is a statistical mechanics toy model illustrating in a simple way some correspondences between number theory and concepts in statistical physics, quantum mechanics, quantum field theory and dynamical systems.

The primon gas IS a quantum field theory (QFT) of a set of non-interacting particles, called the “primons”. It is also named a gas or a free model because the particles are non-interacting. There is no potential. The idea of the primon gas was independently discovered by Donald Spector (D. Spector, Supersymmetry and the Möbius Inversion Function, Communications in Mathemtical Physics 127 (1990) pp. 239-252) and Bernard Julia (Bernard L. Julia, Statistical theory of numbers, in Number Theory and Physics, eds. J. M. Luck, P. Moussa, and M. Waldschmidt, Springer Proceedings in Physics, Vol. 47, Springer-Verlag, Berlin, 1990, pp. 276-293). There have been later works by Bakas and Bowick (I. Bakas and M.J. Bowick, Curiosities of Arithmetic Gases, J. Math. Phys. 32 (1991) p. 1881) and Spector (D. Spector, Duality, Partial Supersymmetry, and Arithmetic Number Theory, J. Math. Phys. 39 (1998) pp.1919-1927) in which it was explored the connection of such systems to string theory.

This model is based on some simple hypothesis:

1st. Consider a simple quantum Hamiltonian, $H$ , having eigenstates $\vert p\rangle$ labelled by the prime numbers “p”.

2nd. The eigenenergies or spectrum are given by $E_p$ and they have energies proportional to $\log p$ . Mathematically speaking,

$H\vert p\rangle = E_p \vert p\rangle$ with $E_p=E_0 \log p$

Please, note the natural emergence of a “free” scale of energy $E_0$ . What is this scale of energy? We do not know!

3rd. The second quantization/second-quantized version of this Hamiltonian converts states into particles, the “primons”. Multi-particle states are defined in terms of the numbers $k_p$ of primons in the single-particle states $p$ :

$|N\rangle = |k_2, k_3, k_5, k_7, k_{11}, \ldots, k_{137},\ldots, k_p \ldots\rangle$

This corresponds to the factorization of $N$ into primes:

$N = 2^{k_2} \cdot 3^{k_3} \cdot 5^{k_5} \cdot 7^{k_7} \cdot 11^{k_{11}} \cdots 137^{k_{137}}\cdots p^{k_p} \cdots$

The labelling by the integer “N” is unique, since every number has a unique factorization into primes.

The energy of such a multi-particle state is clearly

$\displaystyle{E(N) = \sum_p k_p E_p = E_0 \cdot \sum_p k_p \log p = E_0 \log N}$

4th. The statistical mechanics partition function $Z$ IS, for the (bosonic) primon gas, the Riemann zeta function!

$\displaystyle{Z_B(T) \equiv\sum_{N=1}^\infty \exp \left(-\dfrac{E(N)}{k_B T}\right) = \sum_{N=1}^\infty \exp \left(-\dfrac{E_0 \log N}{k_B T}\right) = \sum_{N=1}^\infty \dfrac{1}{N^s} = \zeta (s)}$

with $s=E_0/k_BT=\beta E_0$ , and where $k_B$ is the Boltzmann’s constant and T is the absolute temperature. The divergence of the zeta function at the value $s=1$ (corresponding to the harmonic sum) is due to the divergence of the partition function at certain temperature, usually called Hagedorn temperature. The Hagedorn temperature is defined by:

$T_H=\dfrac{E_0}{k_B}$

This temperature represents a limit beyond the system of (bosonic) primons can not be heated up. To understand why, we can calculate the energy

$E=-\dfrac{\partial}{\partial \beta}\ln Z_B=-\dfrac{E_0}{\zeta (\beta E_0)}\dfrac{\partial \zeta (\beta E_0)}{\partial \beta}\approx \dfrac{E_0}{s-1}$

A similar treatment can be built up for fermions rather than bosons, but here the Pauli exclusion principle has to be taken into account, i.e. two primons cannot occupy the same single particle state. Therefore $m_i$ can be 0 or 1 for all single particle state. As a consequence, the many-body states are labeled not by the natural numbers, but by the square-free numbers. These numbers are sieved from the natural numbers by the Möbius function. The calculation is a bit more complex, but the partition function for a non-interacting fermion primon gas reduces to the relatively simple form

$Z_F(T)=\dfrac{\zeta (s)}{\zeta (2s)}$

The canonical ensemble is of course not the only ensemble used in statistical physics. Julia extended the Riemann gas approach to the grand canonical ensemble by introducing a chemical potential $\mu$ (Julia, B. L., 1994, Physica A 203(3-4), 425), and thus, he replaced the primes p with new primes $pe^{-\mu}$ . This generalisation of the Riemann gas is called the Beurling gas, after the Swedish mathematician Beurling who had generalised the notion of prime numbers. Examining a boson primon gas with fugacity $-1$ , it shows that its partition function becomes

$\overline{Z}_B=\dfrac{\zeta (2s)}{\zeta (s)}$

Remarkable interpretation: pick a system, formed by two sub-systems not interacting with each other, the overall partition function is simply the product of the individual partition functions of the subsystems. From the previous equation of the free fermionic riemann gas we get exactly this structure, and so there are two decoupled systems. Firstly, a fermionic “ghost” Riemann gas at zero chemical potential and, secondly, a boson Riemann gas with energy-levels given by $E(N)=2E_0\ln p_N$ . Julia also calculated the appropriate Hagedorn temperatures and analysed how the partition functions of two different number theoretical gases, the Riemann gas and the “log-gas” behave around the Hagedorn temperature. Although the divergence of the partition function hints the breakdown of the canonical ensemble, Julia also claims that the continuation across or around this critical temperature can help understand certain phase transitions in string theory or in the study of quark confinement. The Riemann gas, as a mathematically tractable model, has been followed with much attention because the asymptotic density of states grows exponentially, $\rho (E)\sim e^E$ , just as in string theory. Moreover, using arithmetic functions it is not extremely hard to define a transition between bosons and fermions by introducing an extra parameter, called kappa $\kappa$ , which defines an imaginary particle, the non-interacting parafermions of order $\kappa$ . This order parameter counts how many parafermions can occupy the same state, i.e. the occupation number of any state falls into the interval $\left[0,\kappa-1\right]$ , and therefore $\kappa=2$ belongs to normal fermions, while $\kappa\rightarrow\infty$ are the usual bosons. Furthermore, the partition function of a free, non-interacting κ-parafermion gas can be defined to be (Bakas and Bowick,1991, in the paper Bakas, I., and M. J. Bowick, 1991, J. Math. Phys. 32(7), 1881):

$Z_\kappa=\dfrac{\zeta (s)}{\zeta (\kappa s)}$

Indeed, Bakas et al. proved, using the Dirichlet convolution $\star$ , how one can introduce free mixing of parafermions with different orders which do not interact with each other

$\displaystyle{f\star g=\sum_{d\vert n}f(d)g\left(\dfrac{n}{d}\right)}$

where the symbol $d\vert n$ means d is a divisor of n. This operation preserves the multiplicative property of the classically defined partition functions, i.e., $Z_{\kappa_1\star \kappa_2}=Z_{\kappa_1}\star Z_{\kappa_2}$ . It is even more intriguing how interaction can be incorporated into the mixing by modifying the Dirichlet convolution with a kernel function or twisting factor

$\displaystyle{f\odot g=\sum_{d\vert n}f(d)g\left( \dfrac{n}{d}\right) K(n,d)}$

Using the unitary convolution Bakas establishes a pedagogically illuminating case, the mixing of two identical boson Riemann gases. He shows that

$Z_\infty\star Z_\infty=\zeta ^2(s)\zeta(2s)=\dfrac{\zeta (s)}{\zeta(2s)}\zeta (s)=Z_2Z_\infty=Z_FZ_B$

This result has an amazing meaning. Two identical boson Riemann gases interacting with each other through the unitary twisting, are equivalent to mixing a fermion Riemann gas with a boson Riemann gas which do not interact with each other. Therefore, one of the original boson components suffers a transmutation/mutation into a fermion gas!

Remark (I): the Möbius function, which is the identity function with respect to the $\star$ operation (i.e. free mixing), reappears in supersymmetric quantum field theories as a possible representation of the $(-1)^F$ operator, where F is the fermion number operator! In this context, the fact that $\mu (n)=0$ for square-free numbers is the manifestation of the Pauli exclusion principle itself! In any QFT with fermions, $(-1)^F$ is a unitary, hermitian, involutive operator where $F$ is the fermion number operator and is equal to the sum of the lepton number plus the baryon number, i.e., $F=B+L$ , for all particles in the Standard Model and some (most of) SUSY QFT. The action of this operator is to multiply bosonic states by 1 and fermionic states by -1. This is always a global internal symmetry of any QFT with fermions and corresponds to a rotation by an angle $2\pi$ . This splits the Hilbert space into two superselection sectors. Bosonic operators commute with $(-1)^F$ whereas fermionic operators anticommute with it. This operator really is, therefore, more useful in supersymmetric field theories.

Remark (II): potential attacks on the Riemann Hypothesis may lead to advances in physics and/or mathematics, i.e., progress in Physmatics!

Remark (III): the energy of the ground state is taken to be zero and the energy spectrum of the excited state is $E(n)=E_0\ln (p_n)$ , where $p_n$ , $n=2,3,5,\ldots$ , runs over the prime numbers. Let N and E denote now the number of particles in the ground state and the total energy of the system, respectively. The fundamental theorem of arithmetic allows only one excited state configuration for a given energy

$E=\ln (n) \;\; mod E_0$

where n is an integer. It immediately means that this gas preserves its quantum nature at any temperature, since only one quantum state is permitted to be occupied. The number fluctuation of any state (even the ground state) is therefore zero. In contrast, the changes in the number of particles in the ground state $\delta n_0$ predicted by the canonical ensemble is a smooth non-vanishing function of the temperature, while the grand-canonical ensemble still exhibits a divergence. This discrepancy between the microcanonical (combinatorial) and the other two ensembles remains even in the thermodynamic limit.

One could argue that the Riemann gas is fictitious/unreal and its spectrum is unrealisable/unphysical. However, we, physicists, think otherwise, since the spectrum $E_N=\ln (N)$ does not increase with N more rapidly than $n^2$ , therefore the existence of a quantum mechanical potential supporting this spectrum is possible (e.g., via inverse scattering transform or supplementary tools). And of course the question is: what kind of system has such an spectrum?

Some temptative ideas for the potential based on elementary Quantum Mechanics will be given in the next section.

11. LOG-OSCILLATORS

Instead of considering the free Riemann gas, we could ask to Quantum Mechanics if there is some potential providing the logarithmic spectrum of the previous section. Indeed, there exists such a potential. Let us factorize any natural number in terms of its prime “atoms”:

$N=p_1^{n_1}p_2^{n_2}\cdots p_m^{n_m}$

Take the logarithm

$\log N=\log \left(p_1^{n_1}p_2^{n_2}\cdots p_m^{n_m}\right)=n_1\log p_1+n_2\log p_2+\ldots+n_m\log p_m$

$\displaystyle{\log N=\sum_{i=1}^{m}n_i\log p_i}$

where $p_i$ are prime numbers (note that if we include “1” as a prime number it gives a zero contribution to the sum).

Now, suppose a logarithmic oscillator spectrum, i.e.,

$\varepsilon_i=\log p_i$ with $p_i=(1),2,3,5,7,11,13,\ldots,137,\ldots,\infty$

with $i=0,1,2,3,4,\ldots,\infty$ . In order to have a “riemann gas”/riemannium, we impose an spectrum labelled in the following fashion

$\varepsilon_s =\log (2s+1)$ $\forall s=0,1,2,3,\ldots,\infty$

Equivalently, we could also define the spectrum of interacting riemannium gas as

$\varepsilon_s=\log (s)$ $\forall s=1,2,3,\ldots,\infty$

In addition to this, suppose the next quantum postulates:

1st. Logarithmic potential:

$V(x)=V_0\ln\dfrac{\vert x\vert}{L}$ with positive constants $V_0, L>0$

From the physical viewpoint, the positive constant $V_0$ means repulsive interaction (force).

2nd. Bohr-Sommerfeld quantization rule:

a) $\displaystyle{I=\dfrac{1}{2\pi}\oint pdx=\hbar \left(s+\dfrac{1}{2}\right)}\; \forall s=0,1,\ldots,\infty$

or equivalently we could also get

b) $\displaystyle{I=\dfrac{1}{2\pi}\oint pdx=\hbar s}\; \forall s=1,2,\ldots,\infty$

3rd. Turning point condition:

$x_s=L\exp \left(\dfrac{\varepsilon_s}{V_0}\right)$

In the case of 2a) we would deduce that

$\displaystyle{\dfrac{\hbar \pi}{2}\left(s+\dfrac{1}{2}\right)=\int_0^{x_s}dx\sqrt{2m\left(\varepsilon_s-V_0\ln \dfrac{x}{L}\right)}}$

$\displaystyle{\dfrac{\hbar \pi}{2}\left(s+\dfrac{1}{2}\right)=\int_0^{x_x}dx\sqrt{-\ln \left(\dfrac{x}{x_s}\right)}=\sqrt{2mV_0}x_s\Gamma \left(\dfrac{3}{2}\right)}$

and then

$x_s=\sqrt{\dfrac{\pi}{2mV_0}}\hbar \left( s+\dfrac{1}{2}\right)$

Then, using the turning point condition in this equation, we finally obtain

$\boxed{\dfrac{\varepsilon_s}{V_0}=\ln (2s+1)+\ln \left(\dfrac{\hbar}{2L}\sqrt{\dfrac{\pi}{2mV_0}}\right)}$ $\forall s=0,1,\ldots,\infty$

In the case of 2b) we would obtain

$\boxed{\dfrac{\varepsilon_s}{V_0}=\ln (s)+\ln \left(\dfrac{\hbar}{L}\sqrt{\dfrac{\pi}{2mV_0}}\right)}$ $\forall s=1,2,\ldots,\infty$

In summary, the logarithmic potential provides a model for the interacting Riemann gas!

12. LOG-POTENTIAL AND CONFINEMENT

Massive elementary particles (with mass m) can be understood as composite particles made of confined particles moving with some energy $pc$ inside a sphere of radius R. We note that we do not define futher properties of the constituent particles (e.g., if they are rotating strings, particles, extended objects like branes, or some other exotic structure moving in circular orbits or any other pattern as trajectory inside the composite particle).

Let us make the hypothesis that there is some force $F$ needed to counteract the centrifugal force $F_c=\dfrac{\kappa c^2}{R}$ . The centrifugal force is equal to $pc/R$ , i.e., the balancing force F is $F=pc/R$ . Then, assuming the two forces are equal in magnitude, we get

$F=F_c=\dfrac{A_1}{R}$

where $A_1$ is some constant, and that equation holds regardless the origin of the interaction. The potentail energy $U$ necessary to confine a constituent particle will be, in that case,

$\displaystyle{U=\int \dfrac{A_1}{R}dR=A_1\int \dfrac{1}{R}dR=A_1\ln \dfrac{R}{R_\star}}$

with $R_\star$ some integration constant to be determined later. The center of mass of the “elementary particle”, truly a composite particle, from the external observer and the mass assinged to the composited system is:

$m=\dfrac{\hbar}{cR}$

The logarithmic potential energy is postulated to be proportional to $m/R$ , and it provides

$U=\dfrac{A_2 m}{R}$

with $A_2$ is another constant. In fact, $A_1, A_2$ are parameters that don’t depend, a priori, on the radius R but on the constitutent particle properties and coupling constants, respectively. Indeed, for instance, we could set and fix the ratio $A_2/A_1$ to the constant $c^2/G_N$ , where $G_N$ is the gravitational constant. However, such a constraint is not required from first principles or from a clear physical reason. From the following equations:

$m=\dfrac{\hbar}{cR}$ and $U=\dfrac{A_2 m}{R}$

we get $\boxed{U=\dfrac{A_2 \hbar}{cR^2}}$

Quantum Mechanics implies that the angular momentum should be quantized, so we can make the following generalization

$U=\dfrac{A_2 m}{cR^2}\rightarrow U_n=\dfrac{A_2 \hbar}{cR_n^2}=\dfrac{A_2 (n+1)\hbar}{cR_0^2}$

$\forall n=0,1,2,\ldots,\infty$

so $R_n^2=\dfrac{R_0^2}{n+1}\leftrightarrow R_n=\dfrac{R_0}{\sqrt{n+1}}$

Using the previous integral and this last result, we obtain

$\ln \left(\dfrac{R_\star}{R_0}\right)=-(n+1)\dfrac{R_\star^2}{R_0^2}$

This is due to the fact that $U_n=A_2\dfrac{\hbar}{cR_n^2}=\dfrac{A_2\hbar (n+1)}{cR_0^2}$ and $U=A_2\ln \dfrac{R}{R_\star}$

Combining these equations, we deduce the value of $R_\star$ as a function of the parameters $A_1,A_2$

$\boxed{R_\star=\sqrt{\dfrac{A_2\hbar}{A_1 c}}}$

The ratio $R_\star/R_0$ can be calculated from the above equations as well, since

$\ln \left(\dfrac{R_\star}{R_0}\right)=-(n+1)\dfrac{R_\star^2}{R_0^2}$ for the case n=0 implies that

$\ln \left(\dfrac{R_\star}{R_0}\right)=-\dfrac{R_\star^2}{R_0^2}$ , and after exponentiation, it yields

$\dfrac{R_\star}{R_0}=e^{-\frac{R_\star^2}{R_0^2}}$

Introducing the variable $x=\dfrac{R_\star}{R_0}$ we have to solve the equation $x=e^{-x^2}$

The solution is $\phi=\dfrac{1}{x}=1.53158$ from which the relationship between $R_\star$ and $R_0$ can be easily obtained. Indeed, we can make more deductions from this result. From $\ln \phi=1/\phi^2$ , then

$R_n=R_\star e^{(n+1)\ln\phi}$

If we take $R_\star=\alpha R_0$ , with $R_0=\hbar/mc$ , then

$\alpha=m_0\sqrt{\dfrac{A_2 c}{A_1\hbar}}$ so

$R_n=R_0e^{K\varphi_n}$ with $K=\dfrac{1}{2\pi}\ln \phi$ and $\varphi_n=2\pi (n+1)+\varphi_s$ $\varphi_s=2\pi \left(\dfrac{\ln \alpha}{\ln \phi}\right)$

Equivalently, the masses would be dynamically generated from the above equations, since

$m_n=\dfrac{\hbar}{R_nc}$ and $m_0=\dfrac{\hbar}{R_0c}$

so we would deduce a particle spectrum given by a logarithmic spiral, through the equation

$\boxed{m_n=m_0e^{K\varphi_n}}$

Remark: The shift $K\rightarrow -K$ implies that the spiral would begin with $m_0$ as the lowest mass and not the biggest mass, turning the spiral from inside to the outside region and vice versa.

In summary, the logarithmic oscillator is also related to some kind of confined particles and it provides a toy model of confinement!

13. HARMONIC OSCILLATOR AND TSALLIS GAS

Is the link between classical statistical mechanics and Riemann zeta function unique or is it something more general? C. Tsallis explained long ago the connection of non-extensive Tsallis entropies an the Riemann zeta function, given supplementary arguments to support the idea of a physical link between Physics, Statistical Mechanics and the Riemann hypothesis. His idea is the following.

A) Consider the harmonic oscillator with spectrum

$E_n=\hbar\omega n$

$E(n),\;\forall n=0,1,2,\ldots,\infty$ , are the H.O. eigenenergies.

B) Consider the Tsallis partition function

$\displaystyle{Z_q (\beta )=\sum_{n=0}^{\infty}e_q^{-\beta E_n}=\sum_{n=0}^{\infty}e_q^{-\beta\hbar\omega n}}$

where $q>1$ and the deformed q-exponential is defined as

$e_q^z\equiv \left[1+(q-1)z\right]_+^{\frac{1}{1-q}}$

and $\left[\alpha\right]=\begin{cases}\alpha, \alpha>0\\ 0,otherwise\end{cases}$

and the inverse of the deformed exponential is the q-logarithm

$\ln_q z=\dfrac{z^{1-q}-1}{1-q}$

It implies that

$\boxed{\displaystyle{Z_q=\sum_{n=0}^{\infty}\dfrac{1}{\left[1+(q-1)\beta\hbar\omega n\right]^{\frac{1}{q-1}}}=\dfrac{1}{\left[(q-1)\beta\hbar \omega\right]^{\frac{1}{q-1}}}\sum_{n=0}^{\infty}\dfrac{1}{\left[\left(\dfrac{1}{(q-1)\beta\hbar\omega}\right)+n\right]^{\frac{1}{q-1}}}}}$

Now, defining the Hurwitz zeta function as:

$\displaystyle{\zeta (s,Q)=\sum_{n=0}^{\infty}\dfrac{1}{\left(Q+n\right)^{s}}=\dfrac{1}{Q^s}+\sum_{n=1}^{\infty}\dfrac{1}{\left(Q+n\right)^{s}}}$

the last equation can be rewritten in a simple and elegant way:

$\boxed{\displaystyle{Z_q=\dfrac{1}{\left[(q-1)\beta\hbar\omega\right]^{\frac{1}{q-1}}}\zeta \left(\dfrac{1}{q-1},\dfrac{1}{(q-1)\beta\hbar\omega}\right)}}$

This system can be called the Tsallis gas or the Tsallisium. It is a q-deformed version (non-extensive) of the free Riemann gas. And it is related to the harmonic oscillator! The issue, of course, is the problematic limit $q\rightarrow 1$ .

In the limit $Q\rightarrow 1$ we get the Riemann zeta function from the Hurwitz zeta function:

$\displaystyle{\zeta (s,1)\equiv \zeta (s)=\sum_{n=1}^{\infty}n^{-s}=\sum_{n=1}^{\infty}\dfrac{1}{n^s}=\prod_{p=2}^{\infty}\dfrac{1}{1-p^{-s}}=\prod_{p}\dfrac{1}{1-p^{-s}}}$

$\displaystyle{\zeta (s)=\dfrac{1}{1-2^{-s}}\dfrac{1}{1-3^{-s}}\ldots\dfrac{1}{1-137^{-s}}\ldots}$

The above equation, the partition function of the Tsallis gas/Tsallisium, connects directly the Riemann zeta function with Physics and non-extensive Statistical Mechanics. Indeed, C.Tsallis himself dedicated a nice slide with this theme to M.Berry:

Remark (I): The link between Riemann zeta function and the free Riemann gas/the interacting Riemann gas goes beyond classical statistical mechanics and it also appears in non-extensive statistical mechanics!

Remark (II): In general, the Riemann hypothesis is entangled to the theory of harmonic oscillators with non-extensive statistical mechanics!

14. TSALLIS ENTROPIES IN A NUTSHELL

For readers not familiarized with Tsallis generalized entropies, I would like to expose you the main definitions of such a generalization of classical statistical entropy (Boltzmann-Gibbs-Shannon), in a nutshell! I have to discuss more about this kind of statistical mechanics in the future, but today, I will only anticipate some bits of it.

Tsallis entropy (and its Statistical Mechanics/Thermodynamics) is based on the following entropy functionals:

1st. Discrete case.

$\boxed{\displaystyle{S_q=k_B\dfrac{1-\displaystyle{\sum_{i=1}^W p_i^q}}{q-1}=-k_B\sum_{i=1}^Wp_i^q\ln_q p_i=k_B\sum_{i=1}^Wp_i\ln_q \left(\dfrac{1}{p_i}\right)}}$

plus the normalization condition $\boxed{\displaystyle{\sum_{i=1}^Wp_i=1}}$

2nd. Continuous case.

$\boxed{\displaystyle{S_q=-k_B\int dX\left[p(X)\right]^q\ln_q p(X)=k_B\int dX p(X)\ln_q\dfrac{1}{p(X)}}}$

plus the normalization condition $\boxed{\displaystyle{\int dX p(X)=1}}$

3rd. Quantum case. Tsallis matrix density.

$\boxed{\displaystyle{S_q=-k_BTr\rho^q\ln _q\rho\equiv k_BTr\rho \ln_q\dfrac{1}{\rho}}}$

plus the normatlization condition $\boxed{Tr\rho=1}$

In all the three cases above, we have defined the q-logarithm as $\ln_q z\equiv\dfrac{z^{1-q}-1}{q-1}$ , $\ln_1 z\equiv \ln z$ , and the 3 Tsallis entropies satisfy the non-additive property:

$\boxed{\dfrac{S_q(A+B)}{k_B}=\dfrac{S_q (A)}{k_B}+\dfrac{S_q (B)}{k_B}+(1-q)\dfrac{S_q (A)}{k_B}\dfrac{S_q (B)}{k_B}}$

15. BEYOND QM/QFT: ADELIC WORLDS

Theoretical physicsts suspect that Physics of the spacetime at the Planck scale or beyond will change or will be meaningless. There, the spacetime notion we are familiarized to loose its meaning. Even more, we could find those changes in the fundamental structure of the Polyverse to occur a higher scales of length. Really, we don’t know yet where the spacetime “emerges” as an effective theory of something deeper, but it is a natural consequence from our current limited knowledge of fundamental physics. Indeed, it is thought that the experimental device making measurements and the experimenter can not be distinguished at Planck scale. At Planck scale, we can not know at this moment how the framework of cosmology and the Hilbert space tool of Quantum Mechanics could be obtained with some unified formalism. It is one of the challenges of Quantum Gravity.

Many people and scientists think that geometry and topology of sub-Planckian lengths should not have any relation with our current geometry or topology. We say and believe that geometry, topology, fields and the main features of macroscopic bodies “emerge” from the ultra-Planckian and “subquantum” realm. It is an analogue to the colours of the rainbow emerging from the atoms or how Thermodynamics emerge from Statistical Mechanics.

There are many proposed frameworks to go beyond the usual notions of space and time, but the p-adic analysis approach is a quite remarkable candidate, having several achievements in its favor.

Motivations for a p-adic and adelic approaches as the ultimate substructure of the microscopic world arise from:

1) Divergences of QFT are believed to be absent with such number structures. Renormalization can be found to be unnecessary.

2) In an adelic approach, where there is no prime with special status in p-adic analysis, it might be more natural and instructive to work with adeles instead a pure p-adic approach.

3) There are two paths for a p-adic/adelic QM/QFT theory. The first path considers particles in a p-adic potential well, and the goal is to find solutions with smoothly varying complex-valued wavefunctions. There, the solutions share certain kind of familiarity from ordinary life and ordinary QM. The second path allows particles in p-adic potential wells, and the goal is to find p-adic valued wavefunctions. In this case, the physical interpretation is harder. Yet the math often exhibits surprising features and properties, and some people are trying to explores those novel and striking aspects.

Ordinary real (or even complex as well) numbers are familiar to everyone. Ostroswski’s theorem states that there are essentially only two possible completions of the rational numbers ( “fractions” you do know very well). The two options depend on the metric we consider:

1) The real numbers. One completes the rationals by adding the limit of all Cauchy sequences to the set. Cauchy sequences are series of numbers whose elements can be arbitrarily close to each other as the sequence of numbers progresses. Mathematically speaking, given any small positive distance, all but a finite number of elements of the sequence are less than that given distance from each other. Real numbers satisfy the triangle inequality $\vert x+y\vert \leq \vert x\vert +\vert y\vert$ .

2) The p-adic numbers. The completions are different because of the two different ways of measuring distance. P-adic numbers satisfy an stronger version of the triangle inequality, called ultrametricity. For any p-adic number is shows

$\vert x+y\vert _p\leq \mbox{max}\{\vert x\vert_p ,\vert y \vert_p\}$

Spaces where the above enhanced triangle inequality/ultrametricity arises are called ultrametric spaces.

In summary, there exist two different types of algebraic number systems. There is no other posible norm beyond the real (absolute) norm or the p-adic norm. It is the power of Mathematics in action.

Then, a question follows inmediately. How can we unify such two different notions of norm, distance and type of numbers. After all, they behave in a very different way. Tryingo to answer this questions is how the concept adele emerges. The ring of adeles is a framework where we consider all those different patterns to happen at equal footing, in a same mathematical language. In fact, it is analogue to the way in which we unify space and time in relativistic theories!

Adele numbers are an array consisting of both real (complex) and p-adic numbers! That is,

$\mathbb{A}=\left( x_\infty, x_2,x_3,x_5,\ldots,x_p,\ldots\right)$

where $x_\infty$ is a real number and the $x_p$ are p-adic numbers living in the p-adic field $\mathbb{Q}_p$ . Indeed, the infinity symbol is just a consequence of the fact that real numbers can be thought as “the prime at infinity”. Moreover, it is required that all but finitely many of the p-adic numbers $x_p$ lie in the entire p-adic set $\mathbb{Z}_p$ . The adele ring is therefore a restricted direct (cartesian) product. The idele group is defined as the essentially invertible elements of the adelic ring:

$\mathbb{I}=\mathbb{A}^\star =\{ x\in \mathbb{A}, \mbox{where}\;\; x_\infty \in \mathbb{R}^{\star} \;\; \mbox{and} \;\; \vert x_p\vert _p=1,\; \mbox{for all but finitely many primes p.}\}$

We can define the calculus over the adelic ring in a very similar way to the real or complex case. For instance, we define trigonometric functions, $e^X$ , logarithms $\log (x)$ and special functions like the Riemann zeta function. We can also perform integral transforms like the Mellin of the Fourier transformation over this ring. However, this ring has many interesting properties. For example, quadratic polynomials obey the Hasse local-global principle: a rational number is the solution of a quadratic polynomial equation if and only if it has a solution in $\mathbb{R}$ and $\mathbb{Q}_p$ for all primes p. Furthermore, the real and p-adic norms are related to each other by the remarkable adelic product formula/identity:

$\displaystyle{\vert x\vert_\infty \prod_p\vert x\vert_p=1}$

and where $x$ is a nonzero rational number.

Beyond complex QM, where we can study the particle in a box or in a ring array of atoms, p-adic QM can be used to handle fractal potential wells as well. Indeed, the analogue Schrödinger equation can be solved and it has been useful, for instance, in the design of microchips and self-similar structures. It has been conjectured by Wu and Sprung, Hutchinson and van Zyl,here http://arXiv.org/abs/nlin/0304038v1 , that the potential constructed from the non-trivial Riemann zeroes and prime number sequences has fractal properties. They have suggested that $D=1.5$ for the Riemann zeroes and $D=1.8$ for the prime numbers. Therefore, p-adic numbers are an excellent method for constructing fractal potential wells.

By the other hand, following Feynman, we do know that path integrals for quantum particles/entities manifest fractal properties. Indeed we can use path integrals in the absence of a p-adic Schrödinger equation. Thus, defining the adelic version of Feynman’s path integral is a necessary a fundamental object for a general quantum theory beyond the common textbook version. However, we need to be very precise with certain details. In particular, we have to be careful with the definition of derivatives and differentials in order to do proper calculations. Indeed we can do it since both, the adelic and idelic rings have a well defined translation-invariant Haar measure

$Dx=dx_\infty dx_2dx_3\cdots dx_p\cdots$ and $Dx^\star=dx_\infty^\star dx_2^\star dx_3^\star\cdots dx_p^\star\cdots$

These measures provide a way to compute Feynman path integrals over adelic/idelic spaces. It turns out that Gaussian integrals satisfy a generalization of the adelic product formula introduced before, namely:

$\displaystyle{\int_{\mathbb{Q}_p}\chi_\infty (ax_\infty^2+bx_\infty)dx_\infty \prod_p \int_{\mathbb{Q}_p}\chi_p (ax_p^2+bx_p)dx_p=1}$

where $\chi$ is an additive character from the adeles to complex numbers $\mathbb{C}$ given by the map:

$\displaystyle{\chi (x)=\chi_\infty (x_\infty)\prod_p \chi_p (x_p)\rightarrow e^{-2\pi ix_\infty}\prod_p e^{2\pi i\{p\}_p}}$

and $\{x_p\}_p$ is the fractional part of $x_p$ in the ordinary p-adic expression for x. This can be thought of as a strong generalization of the homomorphism $\mathbb{Z}/\mathbb{Z}_n\rightarrow e^{2\pi i/n}$ .Then, the adelic path integral, with input parameters in the adelic ring $\mathbb{A}$ and generating complex-valued wavefunctions follows up:

$\displaystyle{K_{\mathbb{A}} (x'',t'';x',t') =\prod_\alpha \int_{(x' _\alpha ,t' _\alpha)}^{(x'' _\alpha ,t'' _\alpha)}\chi_\alpha \left(-\dfrac{1}{h}\int_{t' _\alpha}^{t''_\alpha}L(\dot{q} _\alpha ,q_\alpha ,t_\alpha )dt_\alpha \right) Dq_\alpha}$

The eigenvalue problem over the adelic ring is given by:

$U(t) \psi_\alpha (x)=\chi (E_\alpha (t))\psi_\alpha (x)$

where U is the time-development operator, $\psi_\alpha$ are adelic eigenfunctions, and $E_\alpha$ is the adelic energy. Here the notation has been simplified by using the subscript $\alpha$ , which stands for all primes including the prime at infinity. One notices the additive character $\chi$ which allows these to be complex-valued integrals. The path integral can be generalized to p-adic time as well, i.e., to paths with fractal behaviour!

How is this p-adic/adelic stuff connected to the Riemannium an the Riemann zeta function? It can be shown that ground state of adelic quantum harmonic oscillator is

$\displaystyle{\vert 0\rangle =\Psi_0 (x)=2^{1/4}e^{-\pi x_\infty^2}\prod_p \Omega (\vert x_p\vert_p)}$

where $\Omega \left(\vert x_p \vert _p\right)$ is 1 if $\vert x_p\vert_p$ is a p-adic integer and 0 otherwise. This result is strikingly similar to the ordinary complex-valued ground state. Applying the adelic Mellin transform, we can deduce that

$\Phi (\alpha)=\sqrt{2}\Gamma \left(\dfrac{\alpha}{2}\right)\pi^{-\alpha/2}\zeta (\alpha)$

where $\Gamma, \zeta$ are, respectively, the gamma function and the Riemann zeta function. Due to the Tate formula, we get that

$\Phi (\alpha)=\Phi (1-\alpha)$ .

and from this the functional equation for the Riemann zeta function naturally emerges.

In conclusion: it is fascinating that such simple physical system as the (adelic) harmonic oscillator is related to so significant mathematical object as the Riemann zeta function.

16. STRINGS, FIELDS AND VACUUM

The Veneziano amplitude is also related to the Riemann zeta function and string theory. A nice application of the previous adelic formalism involves the adelic product formula in a different way. In string theory, one computes crossing symmetric Veneziano amplitudes $A(a,b)$ describing the scattering of four tachyons in the 26d open bosonic string. Indeed, the Veneziano amplitude can be written in terms of Riemann zeta function in this way:

$A_\infty (a,b)=g_\infty^2 \dfrac{\zeta (1-a)}{\zeta (a)}\dfrac{\zeta (1-b)}{\zeta (b)}\dfrac{\zeta (1-c)}{\zeta (c)}$

These amplitudes are not easy to calculate. However, in 1987, an amazingly simple adelic product formula for this tachyonic scattering was found to be:

$\displaystyle{A_\infty (a,b)\prod_p A_p (a,b)=1}$

Using this formula, we can compute and calculate the four-point amplitudes/interacting vertices at the tree level exactly, as the inverse of the much simpler p-adic amplitudes. This discovery has generated a quite a bit of activity in string theory, somewhat unknown, although it is not very popular as far as I know. Moreover, the whole landscape of the p-adic/adelic framework is not as easy for the closed bosonic string as the open bosonic strings (note that in a p-adic world, there is no “closure” but “clopen” segments instead of naive closed intervals). It has also been a source of controversy what is the role of the p-adic/adelic stuff at the level of the string worldsheet. However, there is some reasearch along these lines at current time.

Another nice topic is the vacuum energy and its physical manifestations. There are some very interesting physical effects involving the vacuum energy in both classical and quantum physics. The most important effects are the Casimir effect (vacuum repulsion between “plates”) , the Schwinger effect ( particle creation in strong fields) , the Unruh effect ( thermal effects seen by an uniformly accelerated observer/frame) , the Hawking effect (particle creation by Black Holes, due to Black Hole Thermodynamcis in the corresponding gravitational/accelerated environtment) , and the cosmological constant effect (or vacuum energy expanding the Universe at increasing rate on large scales. Itself, does it gravitate?). Riemann zeta function and its generalizations do appear in these 4 effects. It is not a mere coincidence. It is telling us something deeper we can not understand yet. As an example of why zeta function matters in, e.g., the Casimir effect, let me say that zeta function regularizes the following general sum:

$\boxed{\displaystyle{\sum_{n\in \mathbb{Z}}\vert n\vert^d =2\zeta (-d)}}$

Remark: I do know that I should have likely said “the cosmological constant problem”. But as it should be solved in the future, we can see the cosmological constant we observe ( very, very smaller than our current QFT calculations say) as “an effect” or “anomaly” to be explained. We know that the cosmological constant drives the current positive acceleration of the Universe, but it is really tiny. What makes it so small? We don’ t know for sure.

Remark(II): What are the p-adic strings/branes? I. Arefeva, I. Volovich and B. Dravogich, between other physicists from Russia and Eastern Europe, have worked about non-local field theories and cosmologies using the Riemann zeta function as a model. It is a relatively unknown approach but it is remarkable, very interesting and uncommon. I have to tell you about these works but not here, not today. I went too far, far away in this log. I apologize…

17. SUMMARY AND OUTLOOK

I have explained why I chose The Spectrum of Riemannium as my blog name here and I used the (partial) answer to explain you some of the multiple connections and links of the Riemann zeta function (and its generalizations) with Mathematics and Physics. I am sure that solving the Riemann Hypothesis will require to answer the question of what is the vibrating system behind the spectral properties of Riemann zeroes. It is important for Physmatics! I would say more, it is capital to theoretical physics as well.

Let me review what and where are the main links of the Riemann zeta function and zeroes to Physmatics:

1) Riemann zeta values appear in atomic Physics and Statistical Physics.

2) The Riemannium has spectral properties similar to those of Random Matrix Theory.

3) The Hilbert-Polya conjecture states that there is some mysterious hamiltonian providing the zeroes. The Berry-Keating conjecture states that the “quantum” hamiltonian corresponding to the Riemann hypothesis is the corresponding or dual hamiltonian to a (semi)classical hamiltonian providing a classically chaotic dynamics.

4) The logarithmic potential provides a realization of certain kind of spectrum asymptotically similar to that of the free Riemann gas. It is also related to the issue of confinement of “fundamental” constituents inside “elementary” particles.

5) The primon gas is the Riemann gas associated to the prime numbers in a (Quantum) Statistical Mechanics approach. There are bosonic, fermionic and parafermionic/parabosonic versions of the free Riemann gas and some other generalizations using the Beurling gas and other tools from number theory.

6) The non-extensive Statistical Mechanics studied by C. Tsallis (and other people) provides a link between the harmonic oscillator and the Riemann hypothesis as well. The Tsallisium is the physical system obtained when we study the harmonic oscillator with a non-extensive Tsallis approach.

7) An adelic approach to QM and the harmonic oscillator produces the Riemann’s zeta function functional equation via the Tate formula. The link with p-adic numbers and p-adic zeta functions reveals certain fractal patterns in the Riemann zeroes, the prime numbers and the theory behind it. The periodicity or quasiperiodicity also relates it with some kind of (quasi)crystal and maybe it could be used to explain some behaviour or the prime numbers, such as the one behind the Goldbach’s conjecture.

8) A link between entropy, information theory and Riemann zeta function is done through the use of the notion of group entropy. Connections between the Veneziano amplitudes, tachyons, p-adic numbers and string theory arise after the Veneziano amplitude in a natural way.

9) Riemann zeta function also is used in the regularization/definition of infinite determinants arising in the theory of differential operators and similar maps. Even the generalization of this framework is important in number theory through the uses of generalizations of the Riemann zeta function and other arithmetical functions similar to it. Riemann zeta function is, thus, one of the simplest examples of arithmetical functions.

10) There are further links of the Riemann zeta function and “vacuum effects” like the Schwinger effect ( pair creating in strong fields) or the Casimir effect ( repulsive/atractive forces between close objects with “nothing” between them). Riemann zeta function is also related to SUSY somehow, either by the striking similarity between the Dirichlet eta function used in Fermi-Dirac statistics or directly with the explicit relationship between the Möbius function and the $(-1)^F$ operator appearing in supersymmetric field theories.

In summary, Riemann zeta function is ubiquitious and it appears alone or with its generalizations in very different fields: number theory, quantum physics, (semi)classical physics/dynamics, (quantum) chaos theory, information theory, QFT, string theory, statistical physics, fractals, quasicrystals, operator theory, renormalization and many other places. Is it an accident or is it telling us something more important? I think so. Zeta functions are fundamental objects for the future of Physmatics and the solution of Riemann Hypothesis, perhaps, would provide such a guide into the ultimate quest of both Physics and Mathematics (Physmatics) likely providing a complete and consistent description of the whole Polyverse.

Then, the main unanswered questions to be answered are yet:

A) What is the Riemann zeta function? What is the riemannium/tsallisium and what kind of physical system do they represent really? What is the physical system behind the Riemann non-trivial zeroes? What does it mean for the Riemann zeroes arising from the Riemann zeta function generalizations in form of L-functions?

B) What is the Riemann-Hilbert-Polya operator? What is the space over the Riemann operator is acting?

C) Are Riemann zeta function and its generalization everywhere as they seem to be inside the deepest structures of the microscopic/macroscopic entities of the Polyverse?

I suppose you will now understand better why I decided to name my blog as The Spectrum of Riemannium…And there are many other reasons I will not write you here since I could reveal my current research.

However, stay tuned!

Physmatics is out there and everywhere, like fractals, zeta functions and it is full of lots of wonderful mathematical structures and simple principles!

7 Comments on “LOG#050. Why riemannium?”

Javier Matos (@ThMathos) says:

2012/11/09 at 7:57 PM

Uauhh¡¡ A really complex post, but very interesting. I´d like to add my two cents.
It´s a video where Zwiebach(string theory physicist) explains very didactically the zeta function.[Sorry, it´s in spanish]
http://www.videos-star.com/watch.php?video=6RCYIfzztmY
http://www.videos-star.com/watch.php?video=h8ZXLYzOcro

Reply
amarashiki says:

2012/11/10 at 4:06 PM

Reply
amarashiki says:

2012/11/10 at 4:09 PM

The music of prime numbers, by M.Du Satoy.

Reply
Alejandro Rivero says:

2012/11/11 at 12:25 AM

It is surprising (or not) that H. M. Edwards in his monograph “Riemann’s Zeta Function” (a compulsory book for anyone going beyond light introductions to the topic) does not include a review of the Polya/Hilbert conjecture. The book is from 1974, so it seems that it was really a forgotten topic.

Reply
- amarashiki says:
  
  2012/11/11 at 12:58 AM
  
  I own that book, and yes, it is surprising, but only in part. Remember that, as far as I know, the Riemann hypothesis and the Hilbert-Polya approach were not so popular in those times. It was a time where numerical computation and computers were in their early stages and becoming more popular, and also a time were the random matrix theory appeared, or the numerical computation of Riemann zeroes was the main task… You know…Anyway, no one has been able till now of guessing the Riemann operator behind the Hilbert-Polya approach/idea. I have tried myself to learn everything about it, every trial and proposal, older and newer,… My conclusion: the operator has to exist but it must be highly non-trivial, otherwise the Berry-Keating XP conjecture or some modification like some proposal by Connes, Okubo, Sierra-Towsend and others would have succeeded. I have my own suspitions about how to focus the problem, but I am in a point where I need to create “new Mathematics” and you know it is not an easy task for a humble theoretical physicist like me, living between two worlds apart.
joseph palazzo (zaybu) says:

2013/09/27 at 6:50 PM

Can someone help: from the functional equation,
I get ζ(0) = ζ(1), which should be infinite not, -1/2?

Reply
- amarashiki says:
  
  2013/09/27 at 7:52 PM
  
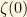  Strictly speaking, you can not apply the functional equation to s=1 and 1-s=1-1=0 because the Riemann zeta function has a pole in s=1 (it is infinite there). The fact that you can calculate $\zeta (1)$ and $\zeta (0)$ is due to the magic of analytic continuation in the whole complex plane, and hence you guess “a regularized” value for an infinite sum like those zeta values or similar results.

The Spectrum of Riemannium

Physmatics in a nutshell, written and explained by a physmatician. Mobilis in mobili!

7 Comments on “LOG#050. Why riemannium?”

Leave a comment Cancel reply

Search box

3QD 2012 award

Read/Watch me here too:

Latest posts

Blog subjects

Parallel reading

Papers, talks, data

Spanish Sci-blogs

Extrasolar planets

Technology

Useful software

Science for Everyone

Images from the Universe

Friends

Fun

Free e-books and lectures

Blogroll

My Linux

E-learning

Blog Statistics

Meta stuff

Tags

My twitter account

TSOR (Facebook)

amarashiki

Physmatics in a nutshell, written and explained by a physmatician. Mobilis in mobili!

LOG#050. Why riemannium?

TABLE OF CONTENTS

Share this:

Related

7 Comments on “LOG#050. Why riemannium?”

Leave a comment Cancel reply

Search box

3QD 2012 award

Read/Watch me here too:

Latest posts

Blog subjects

Parallel reading

Papers, talks, data

Spanish Sci-blogs

Extrasolar planets

Technology

Useful software

Science for Everyone

Images from the Universe

Friends

Fun

Free e-books and lectures

Blogroll

My Linux

E-learning

Blog Statistics

Meta stuff

Tags

amarashiki